Hypothesis
The governing tensors for stress and the rate of deformation can be encoded into a set of independent conjugate base pairs wherein each stress/strain conjugate pair is a scalar pair. Individual constitutive equations govern the response of each base pair, which may be elastic, viscoelastic, etc., as determined from experiment. After the separate constitutive equations for all base pairs have been solved, their constituents can be decoded to update the tensorial states of stress and the rate of deformation. An admissible encoding/decoding algorithm is one-to-one [1].
Milestones
In 2003 Dr. K. R. Rajagopal [2] discussed implicit elastic constitutive models that Helmholtz free energy can be written as a function of temperature, stress and strain. This paper also provides answers either stress causes to strain or strain causes to stress question like most commonly used stress strain relation Hooke’s law ![]() .
.
In 2012 Dr. A. R. Srinivasa [3] explained benefit of upper triangular decomposition for Green elastic materials. With these decomposition researchers don’t need to do tensor invariant calculations to derive constitutive relations. Deformation gradient can be decomposed physically apparent, meaningful and simple elements.
Material Models
Modeling biological fibers
Getting inspiration from these papers, Dr. Freed proposed 1d fiber model [4] which can represents strain limiting behavior. Constitute model can be seen below where ![]() is elastin elastic modulus,
is elastin elastic modulus, ![]() is collegen elastic modulus and
is collegen elastic modulus and ![]() .
.
![]()
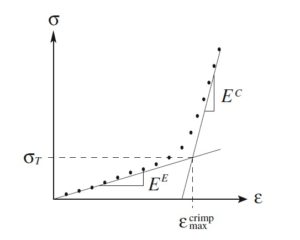
Material parameters are pretty easy to measure by using stress strain curve asymptotes. This method was introduced by Babu [5].
Planar Analysis of Biological Tissues
After upper triangular (QR) factorization, deformation gradient decomposed into rotation tensor and upper triangular matrix. ![]() where
where ![]() is orthogonal matrix and
is orthogonal matrix and ![]() is upper triangular matrix.
is upper triangular matrix.
![]()
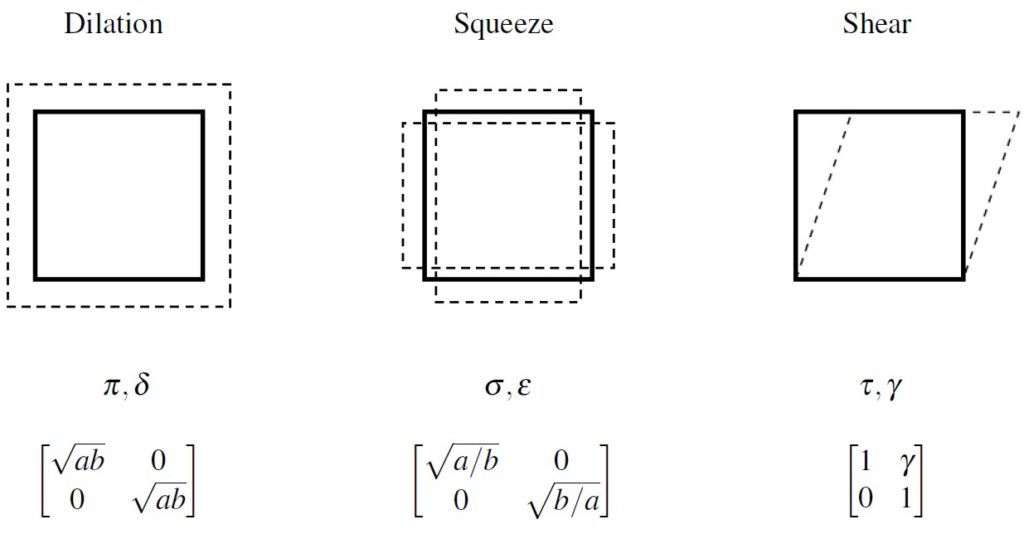
Let’s consider unit square deform into a parallelogram. Extensions ![]() and
and ![]() and shear
and shear ![]() can be directly observed unlike right stretch tensor (U) which comes from polar decomposition.
can be directly observed unlike right stretch tensor (U) which comes from polar decomposition.

Conjugate pair stress/strain approach gives us an opportunity to decompose ![]() into product of three fundamental modes of deformation which are dilation, squeeze and shear in material frame [1] .
into product of three fundamental modes of deformation which are dilation, squeeze and shear in material frame [1] .
Anisotropic Fiber Reinforced Composite Model
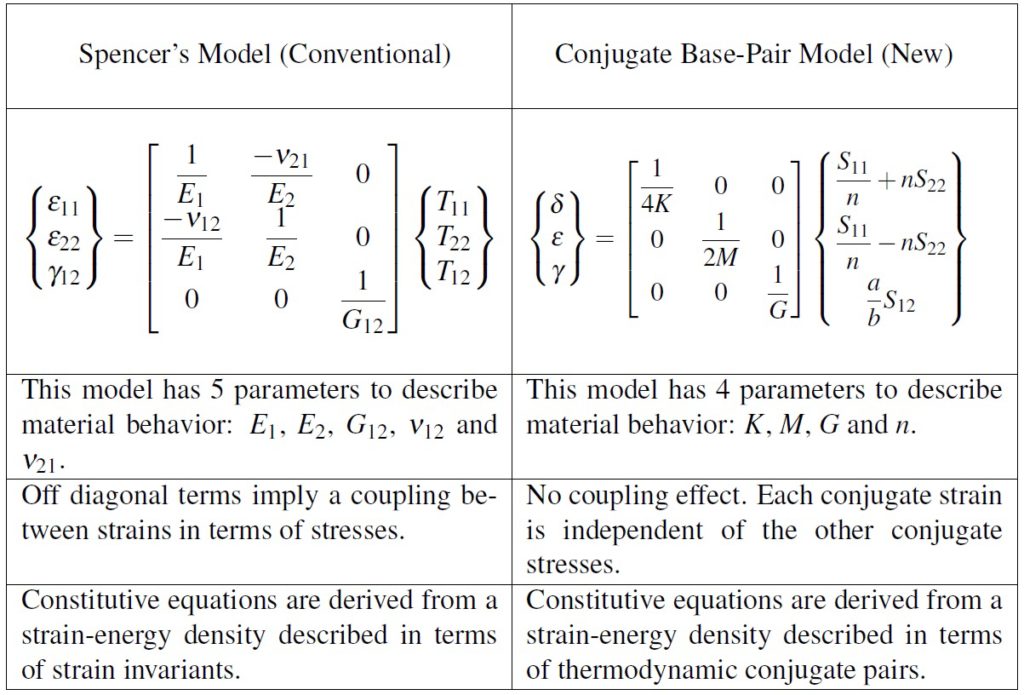
Conjugate pair approach is expanded upon to include anisotropic materials. This model has some advantages over widely used fiber model of Spencer’s [5]. A new parameter n is introduced to quantify the strength of anisotropy. It arises in the decoding/encoding maps, and not in the constitutive equations themselves, which remain isotropic [6]. Differences and similarities between the two models can be seen in the table below.
References
5) Spencer, Anthony James Merrill. “Deformations of fibre-reinforced materials.” (1972): 128.